What is proof by contradiction? How can x be both rational and irrational, please help

A proof by contradiction starts by assuming the opposite of what you're trying to prove. You then work by logical steps until you arrive at a statement that is clearly incorrect (like 1 = 0 or the like), i.e. "a contradiction". If your steps have all been logical then the fault can only be with the assumption at the start, so the original statement must be true.

If x is a particular value it cannot be both rational and irrational. If it is a variable (like in y=mx+c) it can take both rational and irrational values, but not at the same time.

Hey Niko! Basically with a proof of contradiction you start with the original statement, lets say "The square root of 2 is irrational". you start by taking the CONTRADICTION of that, meaning you are going to (try to) prove that the square root of 2 is actually rational. When you try to solve this, you will in fact turn out with the conclusion that the square root of 2 cannot be rationally written down, contradicting your statement of root 2 being rational, hence the original statement of it being irrational is true! Here is a picture, see if this helps!

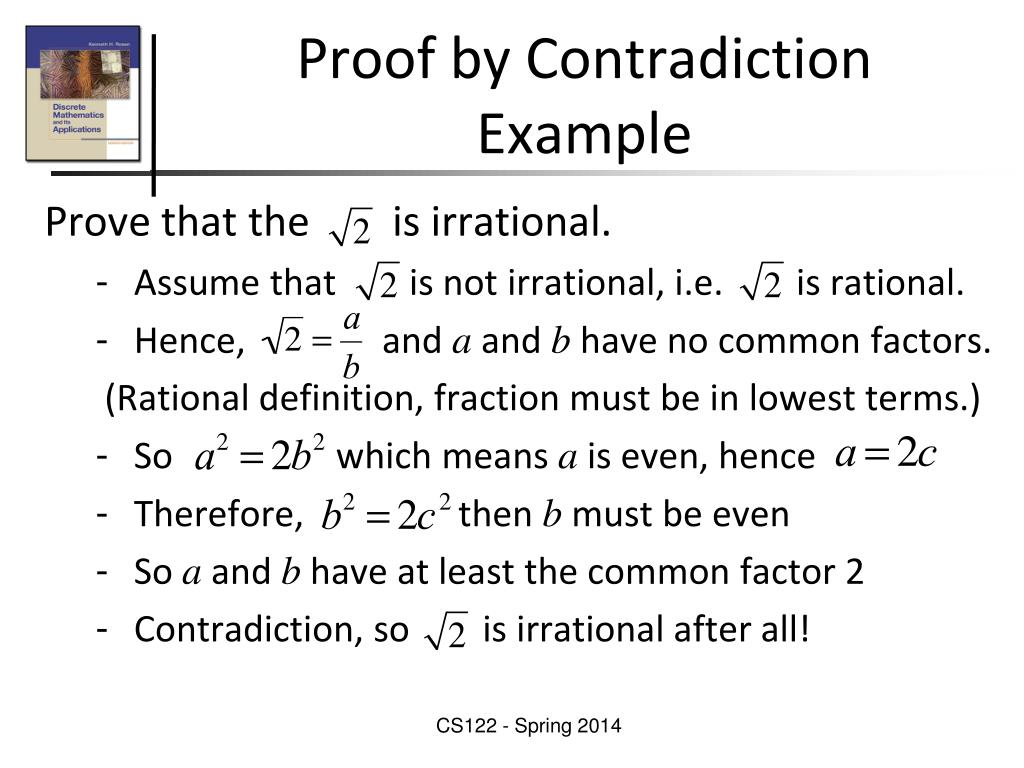
If the questions asks "prove by contradiction that X is irrational", we start by expressing X as an rational number and try showing why this cannot be possible. To do this we'd be contradicting our original statement - "X is an rational number" which means it must be the opposite; an irrational number.

For example, let's say X is the square root of 2.

If they say "Prove by contradiction it's irrational" we must begin by assuming it's the opposite of what they're asking (so that we can "contradict" ourselves later). We can express any rational number as a fraction so we can say if root 2 is rational, we can write it as

√2 = A/B

2 = A^2/B^2

A^2 = 2B^2

This means A^2 is even, since it's 2 multiplied by a number. If this number is k (a placeholder), we can write

A = 2k

Which means our original statement is now

√2 = 2k/B

2 = 4k^2/B^2

2B^2 = 4k^2

B^2 = 2k

Which means B is also even, therefore A/B cannot exist as both A and B are even

This makes our original statement wrong "√2 is rational" as we've treated it as a rational number & it didn't work like the other rational numbers. Therefore it is irrational!

I hope this helps :)

A proof by contradiction is a style of proof where the argument begins by assuming the opposite of what you want to prove. The purpose of doing this is to show that a arguing based on this assumption will result in some absurd, wrong, contradictory or somehow impossible outcome. Arriving at such a result is then taken as evidence that the initial assumption was wrong to begin with, therefore proving that its opposite (which is the thing you wanted to prove in the first place) is true.

A classic example of this type of proof is Euclid's proof that the square root of 2 is an irrational number. He started by assuming that the square root of 2 is rational, and then showed how this assumption leads to a contradictory outcome.

Regarding your second question, a number cannot be simultaneously rational and irrational.

Hi Niko!

In maths, a proof by contradiction is a special (and often very convenient!) type of proof, where you assume the opposite statement (which is not true, but how do you know?), and prove that it leads to a contradiction.

For example, let us do a standard proof, which is that √2 is irrational. For our proof by contradiction, we now assume the opposite statement: √2 is rational. Now the goal is to show that this statement is false.

We can first say that √2 being rational implies that it can be written as a fraction:

√2 = x/y where x and y are coprime, i.e they have no common factor.

Then, it follows that 2 = x²/y² by squaring on both sides. This means that x² = 2y², hence x² is even and naturally x is also even.

Since x is even, it can be written as x=2z, where z is an integer. We remember that we had:

2y² = x²

2y² = (2z)² since x = 2z

2y² = 4z²

y² = 2z²

So y² is even, and again this implies that y is also even. However, if x and y are both even they cannot be coprime!! We have reached a contradiction, which means that the statement √2 is rational is false. In mathematics, this means that the opposite statement is true. Hence we have proved that √2 is irrational (however this does not imply that √2 is both irrational and rational - that's impossible!).

In this case, doing a proof by contradiction is extremely convenient, because it is sometimes easier to prove that something is untrue.

Hopefully this is helpful!

Simon

In proof by contradiction we start from the assumption that for any statement 'P', precisely one of 'P' and 'not P' must be true. Then if we want to show that 'P' holds, we start by imagining that we are in a hypothetical scenario where 'not P' is the case, then use some chain of reasoning to deduce that in such a hypothetical scenario we must have a contradiction. As we do not allow contradictions, our initial assumption that 'not P' is the case must be faulty, and therefore we can conclude that 'P' is the case. In the case that 'P' is the statement 'x is a rational number' we would suppose temporarily that 'x is irrational' and then argue towards some contradiction (such as x being both rational and irrational simultaneously), then conclude that x is indeed rational.

The idea behind a proof by contradiction is to prove that something can't possibily be true by showing that if it were true, we would get a paradox. For example, suppose we wanted to prove that there is no largest number. We would think about what would happen if there was a largest number, let's call it L. Now consider the number L+1. Clearly this is larger than L, but we just said that L was the largest number. Obviously we can't have a largest number and still have a number larger than it. We can therefore conclude that there is no largest number.

Proof by contradiction is a method of proving where you first assume your statement to be false. Then use logical steps to show that indeed the statement is true. As a statement can not be true and false at the same time, we deduce that the statement is true. A classic example is proving that the square root of 2 is irrational. So first you assume it to be rational and use logical steps to deduce that actually, it can't be rational. Therefore we conclude that it is irrational.

If we wish to prove that x is irrational, we can use proof by contradiction. We can assume x to be rational. The idea now is to play around with the algebra and find an error. As this mathematical error exists when x is rational, this means that x must be irrational and has been proven via proof by contradiction.